Conical frustum calculator

r1 =
r2 =
h =
Units:
 
s = 0
V = 0
L = 0
T = 0
B = 0
A = 0
» Show Work
 

r1 = radius 1, r2 = radius 2, h = height, s = slant height, V = volume

L = lateral surface area, T = top surface area, B = base surface area,A = total surface area

A frustum formingan upper base and a lower base that are circular and parallel, It can be formed from a right circular cone by cutting off the tip of the cone.

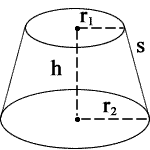
For example, To r1 = 2, r2 = 3, h = 4,

 

  1. To calculate s, use the following formula: √((r1 - r2)2 + h2). When you substitute r1, r2, and h for their values, you get √((2 - 3)2 + 42), which equals 4.12311 m.
  2. To calculate V, use the following formula: (1/3)πh(r12 + r22 + (r1 + r2)). When you substitute r1, r2, and h for their values, you get (1/3)π*4*(22 + 32 + (2 * 3)), which equals 79.58701 m3.
  3. To calculate L, use the following formula: π * (r1 + r2) * s. When you substitute r1, r2, and s for their values, you get π * (2 + 3) * 4.12311, which equals 64.76566 m2.
  4. To calculate T, use the following formula: πr12. When you substitute r1 for its value, you get π * 22, which equals 12.56637 m2.
  5. To calculate B, use the following formula: πr22. When you substitute r2 for its value, you get π * 32, which equals 28.27433 m2.
  6. To calculate A, use the following formula: π(r12 + r22 + (r1 + r2) * s). When you substitute r1, r2, and s for their values, you get π(22 + 32 + (2 + 3) * 4.12311), which equals 105.60636 m2.

 

Buy A Calculator On Amazon
A frustum formingan upper base and a lower base that are circular and parallel, It can be formed from a right circular cone by cutting off the tip of the cone.
Recommendation Calculators Mortgage Affordability Calculator Simple Interest Mortgage Calculator United States (US) Income Tax Calculator UK Income Tax Calculator Self Employment Tax Calculator Sales Tax Calculator Property Millage Tax Calculator Medicare Tax Payment Calculator France Income Tax Calculator Federal Income Tax Calculator Value Added Tax(VAT) Calculator China Income Tax Calculator Australian Income Tax Calculator Interest Only Mortgage Calculator Interest Only Mortgage payment Calculator